Obtaining z_alpha for a Left-tailed Region

If you know $\alpha$ and want the value of $z_{\alpha}$ for a left-tailed region with probability $\alpha$ from a $z$ -distribution, the appropriate formula is:

   =NORMSINV$$(1-\alpha)$$

For example, if $\alpha=0.05$ , the formula is

   =NORMSINV$$(1-0.05)$$

When this command executes, the result is $1.64$ , which is the value of $z_{\alpha}$ when we have $\alpha=0.05$ .

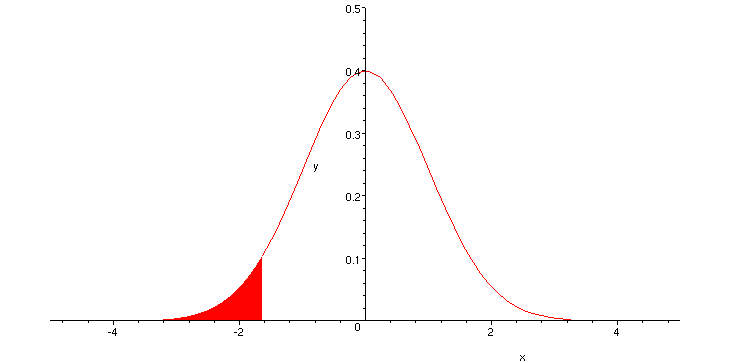
For a Left-tailed test, we are interested in the region from $-\infty$ to $-z_\alpha$ .

The graph below represents this result. The shaded area goes from $-\infty$ to $-1.64$ , and has total area equal to $\alpha$ , or $0.05$ .

Note that we changed the sign of the value that NORMSINV returned. Alternatively, we could have obtained the value $-1.64$ directly by coding $=$NORMSINV$(0.05)$ .

This threshold value would be used to construct a Left-tailed test or confidence region.

(Note: this is the correct syntax for Excel. It is slightly different for other spreadsheets. Consult the help menu of the spreadsheet you are using to find the correct syntax for your spreadsheet program).