Computational Details

Under the null hypothesis, the proportion of individuals in the population that have the characteristic is $p_0$ .

Under the assumptions that $n\cdot p_0\cdot(1-p_0)\geq10$ and $n\leq0.05N$ , the population standard deviation is:

$$\sigma\;=\;\sqrt{p_0\cdot(1-p_0)}$$

and the data are approximately distributed normally with mean $p_0$ and standard deviation $\sqrt{p_0\cdot(1-p_0)}$ .

With this assumption, if the null hypothesis is true, the sample proportion

$$\hat{p}\;=\;\frac{x}{n}$$

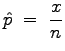
will be approximately normally distributed with the following parameters:

   mean$$\;=\;p_0$$   standard deviation$$\;=\;\sqrt{\frac{p_0\cdot(1-p_0)}{n}}$$

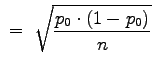
The strategy for testing the hypothesis is to consider $\hat{p}$ to be a single observation from this distribution, standardize it based on the assumption that its mean is $p_0$ and its standard deviation is $\sqrt{p_0(1-p_0)/n}$ , and base our decision to accept or reject the null hypothesis on where the standardized value or $Z$ -score falls on the standard normal bell curve.

We calculate the standardized or $Z$ -score for the sample proportion $\hat{p}$ as:

$$Z\;=\;\frac{\hat{p}-p_0}{\sqrt{\frac{p_0\cdot(1-p_0)}{n}}}$$

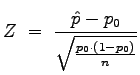
(This value is in cell B13)

The exact decision rule to accept or reject the null hypothesis depends on whether we want:

• a two-tailed test $(p\neq p_0)$
• a left-tailed $(p < p_0)$
• a right-tailed test. $(p > p_0)$

See the sections below for details on these three cases.